Hydria Virtual Museum: The aqueduct of Eupalinos at Samos

Waterworks

The aqueduct was built to improve the water capacity of the city and its then inhabitants and to ensure this supply even in a state of siege.

A spring with a daily supply of 400m³ was located behind the mountain Ambelos in the north-west of the city, at an altitude of 52 meters. The possible options for the water to reach the city were either around the mountain, with a surface pipe, or through the mountain with a tunnel.

Eupalinos chose the second solution, that would guarantee enough water supply inside the city walls even in the case of siege.

In its total length the aqueduct covered a distance of over 2.3 km and was comprised of three parts:

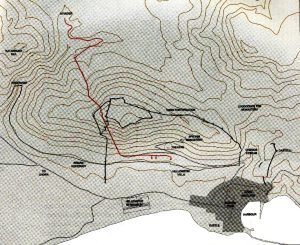
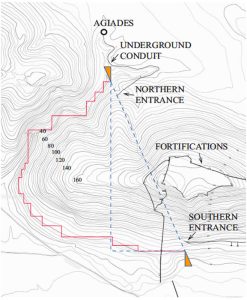
A. The “great spring” (todays’ Agiades) as mentioned by Herodotus, with daily yield of 400m³ lied north-west, outside the city walls and behind the height of mountain Ambelos, later known as Castron. Partially cut into stone, a large reservoir with an horizontal roof supported by at least fifteen strong stone pillars was built. The reservoir remains in good condition nowadays, underneath the church of St. John, while the water is still channelled to Pythagoreion.

From the spring’s reservoir an underground conduit 890 m long and 0,60 – 0,70 m wide, was dug winding along the natural curves to reach the northern tunnel entrance. Vertical, periodic shafts, every 30-50 m, were employed to guide the route of the conduit (qanat technique).

B. The most challenging part of the aqueduct and probably the first to be constructed was the second part; the tunnel attempting to cross the mountain Ambelos at a height 55 m above sea level and 180 m below the mountain top, without giving away the water route to possible enemies of Samos city.

C. Leaving the tunnel, a subterranean conduit of 520 m was the third part of the aqueduct -similar to the first part-, constructed by connecting shafts parallel to the foot of the mountain, at distances varying from 11 to 25m, visible on the surface. Water was channelled from the southern exit eastwards into the town of Pythagoreion.

Although the end point of the aqueduct has not yet been located, a distribution system should have fed the fountains of the city (at least two have been recovered, of monumental dimensions), probably leading the water as far as the harbour.

The second part of the aqueduct, the famous Eupalinos’ tunnel is further analysed below.

Tunnel description

As mentioned above, the tunnel was hewn through the limestone rock of the mountain. Having a total length 1.036 m and average dimensions (H x W) 1,8 m x 1,8m it took roughly 10 years to be constructed.

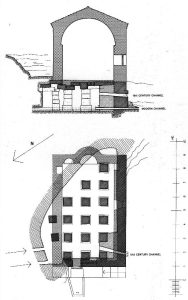
The tunnel floor was horizontal to prevent groundwater from gathering at the workface, while the spring water flowed in a slightly sloping rectangular channel / ditch (0,7m width, inclination: 0,45%), excavated along one side of the tunnel floor. This channel/ditch in which the water was flowing, was probably dug after the two parts of the horizontal tunnel had successfully met. It starts at a depth of approximately 2m (northern entrance) and gradually reaches 9m at its exit. Inside this channel 5.000 clay water pipes were laid with a diameter of 0,26m. The channel is today covered with railings for safety reasons.

The roof of the tunnel was reinforced with stone masonry (total length: 165m) in the places that the rock was dangerously loose. Through this, apart from the archaic, polygonal masonry with pointed roof, a roman repairing phase could be also recognised, employing walls of small stones with plaster and barrel-vaulted roof. Later interventions are dated in the Byzantine times, when the tunnel was used as a refuge, before being abandoned.

In total, some 7.000 cubic meters of rock were excavated to complete the tunnels of this hydraulic feat, using only picks, hammers, and chisels.

Tunnel design

A number of critical decisions were taken at the beginning of the feat, including:

a. its straight direction and level ground,
b. the altitude: 55,8m in order to minimise excavation works,
c. starting to work from both ends in order to save time.

Considering these, several propositions were made on the intellectual feat of hewing the tunnel, without any compasses or topographic maps at their disposal, using only principles of geometry to be codified only several centuries later.

The exact theoretical method employed to build the tunnel still remains a question, although research (Kienast 1995) favours the principles of Hero of Alexandria, an ancient Greek mathematician and engineer (10-70 AD). It is believed that the engineer measured the distance around the mountain using right triangles: distance moving west was subtracted from the distance moving east to determine one side of a right triangle. The sum of all the north-south distances equalled the length of the second side of the triangle, while the line connecting the two, the hypotenuse of the triangle, was actually the route of the proposed tunnel. Once these lines were calculated, even buried beneath the mountain, two similar, right triangles would have been created in each side of the tunnel to direct the route of the excavation.

Tunnel execution

One of the many topographic difficulties Eupalinos faced was the determination of the altitude for the entrance and the exit of the tunnel on the slopes of the mountain. To do this he first marked by wooden posts a route on the top of the mountain, the projected route of the envisaged tunnel. The route of the tunnel should have been inspected in relation to this trace marked by the wooden posts and used as a paradigm for both sides.

Another challenge was not to deviate from the horizontal level during the process of the excavation and Eupalinos probably employed a chorobates, an ancient leveller and a dioptra. For the measuring of distances, in straight lines and angles diabetesand goniometers were probably used. Eupalinos used a unit of 20.59 meters for distance measurements and 7.5 degrees (1/12 of a right angle) for setting out directions.

A number of measure marks are still visible on the walls of the tunnel in red paint!

In the beginning of the project, in order to test the accuracy of his calculations Eupalinos, opened a vertical shaft about 40 meters after the entrance of the northern part of the tunnel. The shaft that was dug as extension of one of the wooden posts accurately coincided the below tunnel and confirmed his estimates.

This description would end, if after about 200 meters from its northern mouth Eupalinos had not deviated from the straight line. The excavation labourers encountered problems in the geological strata, probably a combination of loose rock and water. Eupalinos was forced to make a diversion firstly westwards by 25 degrees and after 150 m eastwards, forming the two sides of a right triangle whose hypotenuse would have been the original route.

The diversion however continued after reaching it, probably to locate solid rock, moving in a crooked line towards the south branch of the tunnel. This had been finished two years before in an eastward angle, after 390 meters from the southern mouth of the tunnel. This important extension, a feat vital for the conclusion of the project, was marked by the engineer with the word ΠΑΡΑΔΕΓΜΑ (= additional stretch) on the eastern wall of the tunnel, reflecting his pride and giving a hint to the possible researchers that would try to decipher the enigma of the tunnel design and execution.

Nearing the meeting point the northern crew changed direction in a sharp angle eastwards, to avoid missing the southern part. In addition to that, in the last meters before the rendezvous Eupalinos raised the ceiling of the north branch by 2.5m, while the floor of the south has been lowered by 0.6m, providing a catching height of almost 5m.

These were unnecessary precautions since at the meeting point, almost under the summit of the mountain, the errors were minimal: the horizontal error was 0,7m and the vertical 0,04m!

Next: PEOPLE / CULTURE